(Translated by https://www.hiragana.jp/)
Models based on Mittag-Leffler functions for anomalous relaxation in dielectrics | The European Physical Journal Special Topics Skip to main content
SpringerLink
Log in

Models based on Mittag-Leffler functions for anomalous relaxation in dielectrics

  • Modelling
  • Regular Article
  • Published:
The European Physical Journal Special Topics Aims and scope Submit manuscript

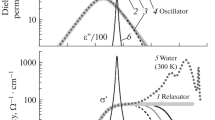
Abstract.

We revisit the Mittag-Leffler functions of a real variable t, with one, two and three order-parameters {αあるふぁ,βべーた,γがんま}, as far as their Laplace transform pairs and complete monotonicity properties are concerned. These functions, subjected to the requirement to be completely monotone for t > 0, are shown to be suitable models for non–Debye relaxation phenomena in dielectrics including as particular cases the classical models referred to as Cole–Cole, Davidson–Cole and Havriliak–Negami. We show 3D plots of the relaxations functions and of the corresponding spectral distributions, keeping fixed one of the three order-parameters.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. K.S. Cole, R.H. Cole, J. Chem. Phys. 9, 341 (1941)

    Article  ADS  Google Scholar 

  2. K.S. Cole, R.H. Cole, J. Chem. Phys. 10, 98 (1942)

    Article  ADS  Google Scholar 

  3. D.W. Davidson, R.H. Cole, J. Chem. Phys. 19, 1484 (1951)

    Article  ADS  Google Scholar 

  4. K. Diethelm, An Application-Oriented Exposition Using Differential Operators of Caputo Type, Springer Lecture Notes in Mathematics No 2004 (Springer, Berlin, 2010)

  5. G. Gripenberg, S.O. Londen, O.J. Staffans, Volterra Integral and Functional Equations (Cambridge University Press, Cambridge, 1990), p. 143

  6. H. Hilfer (ed.), Applications of Fractional Calculus in Physics (World Scientific, Singapore, 2000)

  7. A. Hanyga, M. Seredyńska, J. Stat. Phys. 131, 269 (2008)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  8. S. Havriliak Jr., S. Negami, J. Polymer Sci. C 14, 99 (1966)

    Google Scholar 

  9. S. Havriliak, S. Negami, Polymer 8, 161 (1967)

    Article  Google Scholar 

  10. A.K. Jonscher, Dielectric Relaxation in Solids (Chelsea Dielectrics Press, London, 1983)

  11. H. Hilfer, J. Non-Cryst. Solids 305, 122 (2002)

    Article  ADS  Google Scholar 

  12. H. Hilfer, Phys. Rev. E 65, 061510/1 (2002)

    Article  ADS  Google Scholar 

  13. A.K. Jonscher, Universal Relaxation Law (Chelsea Dielectrics Press, London, 1996)

  14. F. Mainardi, Fractional Calculus and Waves in Linear Viscoelasticity (Imperial College Press, London, 2010)

  15. A. Jurlewicz, K. Weron, J. Non-Cryst. Solids 305, 112 (2002)

    Article  ADS  Google Scholar 

  16. A. Jurlewicz, K. Weron, M. Teuerle, Phys. Rev. E 78, 011103/1 (2008)

    Article  ADS  Google Scholar 

  17. A.M. Mathai, R.K. Saxena, H.J. Haubold, The H Function, Theory and Applications (Springer, Amsterdam, 2006)

  18. I. Podlubny, Fractional Differential Equations (Academic Press, San Diego, 1999)

  19. F. Mainardi, R. Gorenflo, Fract. Calculus Appl. Anal. 10, 269 (2007) [E-print http://arxiv.org/abs/0801.4914]

    MathSciNet  MATH  Google Scholar 

  20. A.H. Zemanian, Realizability Theory for Continuous Linear Systems (Academic Press, San Diego, 1972)

  21. K.S. Miller, S.G. Samko, Real Anal. Exchange 23, 753 (1997)

    MathSciNet  MATH  Google Scholar 

  22. K.S. Miller, S.G. Samko, Integr. Trans. Spec. Funct. 12, 389 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  23. V.V. Novikov, K.W. Wojciechowski, O.A. Komkova, T. Thiel, Mater. Sci. Poland 23, 977 (2005)

    Google Scholar 

  24. I. Podlubny, Fractional Differential Equations (Academic Press, San Diego, 1999)

  25. H. Pollard, Bull. Amer. Math. Soc. 54, 1115 (1948)

    Article  MathSciNet  MATH  Google Scholar 

  26. T.R. Prabhakar, Yokohama Math. J. 19, 7 (1971)

    MathSciNet  MATH  Google Scholar 

  27. W.R. Schneider, Expositiones Math. 14, 3 (1996)

    MATH  Google Scholar 

  28. R.T. Sibatov, D.V. Uchaikin [E-print: arXiv:1008.3972] 5 pages

  29. B. Szabat, K. Weron, P. Hetman, J. Non-Cryst. Solids 353, 4601 (2007)

    Article  ADS  Google Scholar 

  30. A. Stanislavsky, K. Weron, J. Trzmiel, Eur. Phys. Lett. (EPL) 91, 40003/1 (2010)

    ADS  Google Scholar 

  31. A.H. Zemanian, Realizability Theory for Continuous Linear Systems (Academic Press, San Diego, 1972)

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, IMECC, University of Campinas, 13083-869 Campinas, SP, Brazil

    E. Capelas de Oliveira & J. Vaz Jr.

  2. Department of Physics, University of Bologna, via Irnerio 46, 40126, Bologna, Italy

    F. Mainardi

Authors
  1. E. Capelas de Oliveira
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. F. Mainardi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. J. Vaz Jr.
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to E. Capelas de Oliveira, F. Mainardi or J. Vaz Jr..

Rights and permissions

Reprints and permissions

About this article

Cite this article

de Oliveira, E., Mainardi, F. & Vaz, J. Models based on Mittag-Leffler functions for anomalous relaxation in dielectrics. Eur. Phys. J. Spec. Top. 193, 161–171 (2011). https://doi.org/10.1140/epjst/e2011-01388-0

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1140/epjst/e2011-01388-0

Keywords

Search

Navigation