Rectangle | Definition, Properties, Formulas
A rectangle is a quadrilateral with four sides and following properties.
- All four angles are right angles (90°).
- The opposite sides of a rectangle are equal in length and parallel to each other.
- A rectangle is a two-dimensional flat shape.
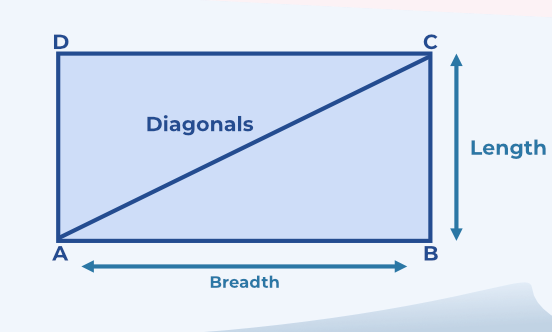
Illustration of a Rectangle
Here, sides AB and CD are equal and parallel and are called the lengths of the rectangle. Also, the sides BC and AD are called the breadth of the rectangle and they are also parallel and equal. AC is the diagonal of the rectangle.
Read More: Quadrilateral
Length and Breadth of Rectangle
The two sides of the rectangle are classified as the Length and two sides are classified as Breadth.
The longer side of the rectangle is called the length of the rectangle and the shorter side of the rectangle is called the breadth of the rectangle.
From the above image, we have
- Length of the Rectangle ABCD = AB and CD
- Breadth of the Rectangle ABCD = BC and DA
The length and the breadth of the rectangle are used to find the Area, Perimeter, and Length of the Diagonal of the Rectangle.
Diagonal of Rectangle
Diagonal of the rectangle is the line connecting the two points of the rectangle by skipping one point.
In Figure 1 the rectangle ABCD has the diagonal AC and its length can be calculated using the Pythagoras theorem. The diagonal of the rectangle are equal and they bisect each other.
Diagonal of Rectangle Formula
If the length and the breadth of the rectangle are l and b respectively then the diagonal of the rectangle is
d = √( l2 + b2)
Rectangle Properties
Some of the important properties of a rectangle are:
- Rectangle is a Polygon with four sides.
- The angle formed by adjacent sides is 90°.
- Rectangle is a Quadrilateral.
- Opposite sides of a Rectangle are Equal and Parallel.
- Rectangle is considered to be a Parallelogram.
- Interior Angles of a Rectangle are equal and the value of each angle is 90°.
- Sum of all Interior Angles is 360°.
- Diagonals of a Rectangle are equal.
- Diagonals of a Rectangle bisect each other.
- Length of the diagonal is found by Pythagoras theorem. If the length and the breadth of the rectangle are l and b respectively then the diagonal of the rectangle is d = √( l2 + b2).
Rectangle Formula
A rectangle is a closed quadrilateral in which the sides are equal and parallel, there are various formulas that are used to find various parameters of the rectangle.
Some of the important formulas of the rectangle are,
- Perimeter of Rectangle
- Area of Rectangle
| Property | Formula |
|---|---|
| Area | Length × Breadth |
| Perimeter | 2 × (Length + Breadth) |
Let’s discuss them in some detail.
Rectangle Perimeter
Perimeter of the rectangle is defined as the total length of all the sides of the rectangle. It is also called the circumference of the rectangle.
It is measured in units of length, i.e. m, cm, etc.
Perimeter of Rectangle Formula
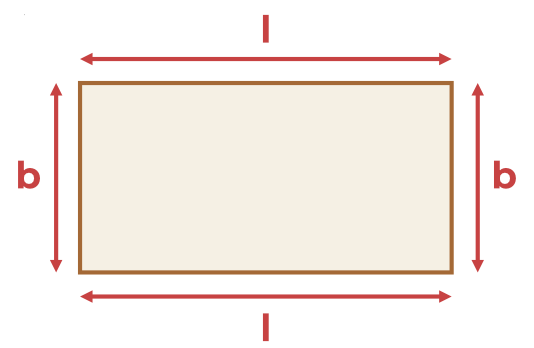
In a rectangle, if the length(l) and breadth(b) are given then the perimeter of the rectangle is found using the formula,
Perimeter of Rectangle = 2 (l + b)
their
Where,
- l is the length of the rectangle.
- b is the breadth of the rectangle.
The figure representing the perimeter of the rectangle is,
Perimeter of Rectangle Illustration
Length of Rectangle Formula
In a rectangle, if the breadth(b) and perimeter(P) are given then its length(l) is found using,
(length) l = (P / 2) – b
In a rectangle, if the breadth(b) and area (A) are given then its length(l) is found using,
(length) l = A/b
Formula for Breadth of Rectangle
In a rectangle, if the length(l) and perimeter(P) are given then its breadth(b) is found using,
(breadth) b = (P / 2) – l
In a rectangle, if the length(l) and area (A) are given then its breadth(b) is found using,
(breadth) b = A/l
Rectangle Area
Area of the rectangle is defined as the total space occupied by the rectangle. It is the space inside the boundary or the perimeter of the rectangle.
The area of the rectangle is dependent on the length and breadth of the rectangle, it is the product of the length and the breadth of the rectangle. The area of a rectangle is measured in square units, i.e. in m2, cm2, etc.
Area of Rectangle Formula
In a rectangle, if the length(l) and breadth(b) are given then the area of the rectangle is found using the formula,
Area of Rectangle = l × b
where,
- l is the length of the rectangle
- b is the breadth of the rectangle
Here is a diagram representing the area of the rectangle is,

Area of Rectangle Diagram
Types of Rectangle
Rectangles are of two types, which are:
- Square
- Golden Rectangle
Let’s learn about them in detail.
Square
A square is a special case of a rectangle in which all the sides are also equal. It is a quadrilateral in which opposite sides are parallel and equal, so it can be considered a rectangle.
The diagram of a square is shown below,
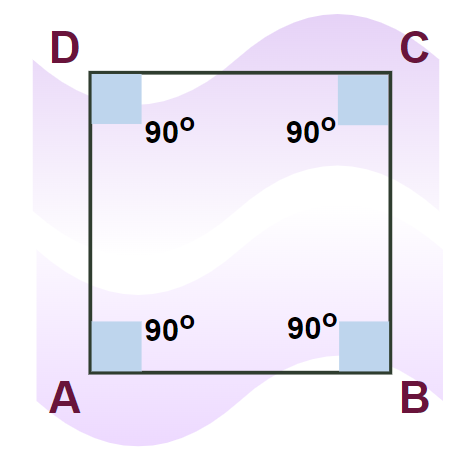
Diagram of a Square
Learn More: Square
Golden Rectangle
A rectangle in which the ‘length to the width’ ratio is similar to the golden ratio, i.e. equal to the ratio of 1: (1+⎷5)/2 is called the golden rectangle.
They are in the ratio of 1: 1.618 thus, if its width is 1 m then its length is 1.168 m.
The diagram of a Golden Rectangle is shown below,

Must Read
Examples on Rectangle
Here are some solved examples on the basic concepts of rectangle for your help.
Example 1: Find the area of the rectangular photo frame whose sides are 8 cm and 6 cm.
Solution:
Given,
- Length of Photo Frame = 8 cm
- Breadth of Photo Frame = 6 cm
Area of Photo Frame = Length × Breadth
= 6 × 8 = 48 cm2Thus, the area of the photo frame is 48 cm2.
Example 2: Find the perimeter of the rectangular field whose sides are 9 m and 13 m.
Solution:
Given,
- Length of Rectangular Field = 9 m
- Breadth of Rectangular Field = 13 m
Perimeter of Rectangular Field = 2 × (l + b)
= 2 × (9 + 13) = 2 × (22)
= 44 mThus, the perimeter of the rectangular field is 44 m.
Example 3: Find the area and the perimeter of the room with a length of 12 feet and a breadth of 8 feet.
Solution:
Given,
- Length of room (l) = 12 feet
- Breadth of room (b) = 8 feet
Area of Room = Length × Breadth
= 12 × 8 = 96 feet2Perimeter of Room = 2 × (l + b)
= 2 × (12 + 8) = 2 × 20
= 40 feetThus, the area of the room is 96 feet2, and the perimeter is 40 feet.
Example 4: Find the length of the diagonal of the rectangle whose sides are 6 cm and 8 cm.
Solution:
Given,
- Length of rectangle (l) = 6 cm
- Breadth of rectangle (b) = 8 cm
Length of diagonal (d) = √( l2 + b2)
d = √( 82 + 62)
d = √ (64 + 36) = √(100)
d = 10 cmThus, the length of the rectangle is 10 cm



