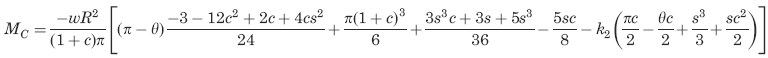Related Resources: calculators

Circular Ring Stress Analysis no. 11 Roarks Formulas for Stress and Strain Equations and Calculator

Circular Ring Moment, Hoop Load, and Radial Shear Equations and Calculator #11.

Per. Roarks Formulas for Stress and Strain Formulas for Circular Rings Section 9, Reference, loading, and load terms #11.
Formulas for moments, loads, and deformations and some selected numerical values.

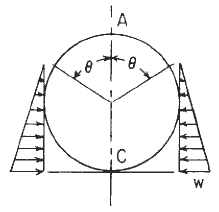Circular Ring Loading #11	Circular Ring Dimensional Properties
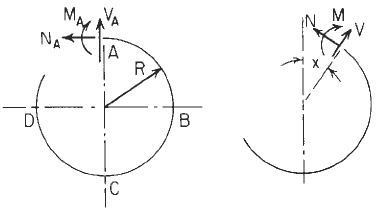Resultant moment, hoop load, and radial shear

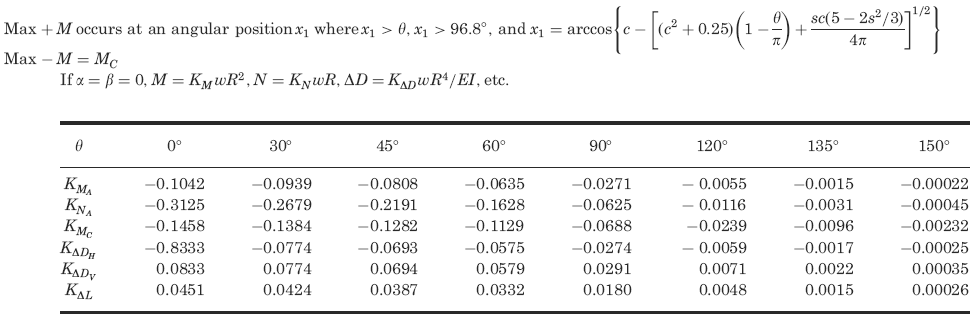
Preview: Circular Ring Moment, Hoop Load, and Radial Shear Calculator #11

General formulas for moment, hoop load, radial shear and deformations.

θ ≥ π / 2

Moment
M = M_A - N_A R ( 1 - u) + V_A R z + LT_M

Hoop Stress
N = N_A u + V_a z + LT_N

Radial Shear
V = - N_A z + V_A u + LT_v

LT_M LT_N, and LT_V are load terms for several types of load.
Note: Loads beyond 180° not support in load terms equations.

LT_M = - w R² / 2 ( c - u )² ⟨ x - θ ⟩⁰

LT_N = w R u ( c - u ) ⟨ x - θ ⟩⁰

LT_v = - w R z ( c - u ) ⟨ x - θ ⟩⁰

Unit step function defined by use of ⟨ ⟩, See explanation at bottom of page.
⟨ x - θ ⟩⁰

V_A = 0

R_i / t ≥ 10 means thin wall for pressure vessels (per. ASME Pressure Vessel Code)

Hoop Stress Deformation Factor α

α = e / R for thick rings
α = I / AR² for thin rings
A = π [ R² - R_i² ]

Transverse (radial) shear deformation factor β

β = 2F (1 + v) e / R for thick rings
β = FEI / GAR² for thin rings

k₁ = 1 - α + β

k₂ = 1 - α

Plastic section modulus
F = Z / I c
Z = Z_x = Z_y = 1.33R³
I_x = I_y = ( π / 4 ) ( R⁴ - R_i⁴)

Deformation in the Horizontal Axis

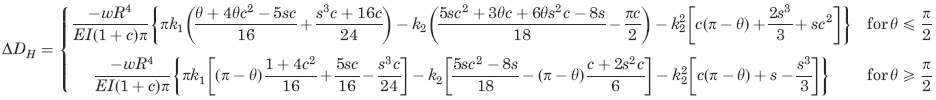
Deformation in the Vertical Axis

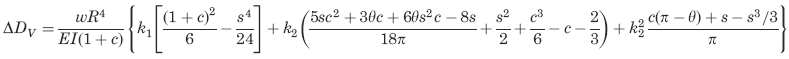
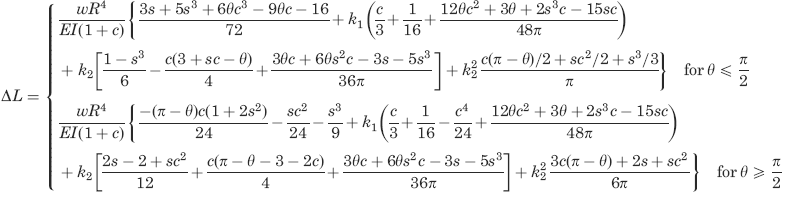
Change of length of Circular Ring

Where (when used in equations and this calculator):

W = load (force);
v and w = unit loads (force per unit of circumferential length);
G = Shear modulus of elasticity
F = Shape factor for the cross section (= Z / I c)
Z = Plastic section modulus
w and v = unit loads (force per unit of circumferential length);
ρ = unit weight of contained liquid (force per unit volume);
M_o = applied couple (force-length);
M_A, M_B, M_c, and M are internal moments at A;B;C, and x, respectively, positive as shown.
N_A N, V_A, and V are internal forces, positive as shown.
E = modulus of elasticity (force per unit area);
v = Poisson’s ratio;
A = cross-sectional area (length squared);
R = Outside radius to the centroid of the cross section (length);
R_i = Inside radius to the centroid of the cross section;
t = wall thickness
I = area moment of inertia of ring cross section about the principal axis perpendicular to the plane of the ring (length⁴). [Note that for a pipe or cylinder, a representative segment of unit axial length may be used by replacing EI by Et³ / 12 (1 - v² )
e ≈ I / ( R A) positive distance measured radially inward from the centroidal axis of the cross section to the neutral axis of pure bending
θ, x, and Φ are angles (radians) and are limited to the range zero to π for all cases except 18 and 19
s = sin θ
c = cos θ
z = sin x,
u = cos x
n = sin Φ
m = cos Φ
α - Hoop stress deformation factor
ΔD_V = Change in vertical diameter (in, mm),
ΔD_H = Change in horizontal diameter (in, mm),
ΔL = Change in lower half of vertical diameterthe vertical motion realative to point C of a line connecting points B and D on the ring,
ΔL_W = Vertical motion relative to point C of a horizontal line connecting the load points on the ring,
ΔL_WH = Change in length of a horizontal line connecting the load points on the ring,
ψ = angular rotation (radians) of the load point in the plane of the ring and is positive in the direction of positive θ.

Unit step function defined by use of ⟨ ⟩
⟨ x - θ ⟩⁰

if x < 0, ⟨ x - θ ⟩⁰ =0;
if x > 0, ⟨ x - θ ⟩⁰ =1;

At x = θ the unit step function is undefined just as vertical shear is undefined directly beneath a concentrated load. The use of the angle brackets ⟨ ⟩ is extended to other cases involving powers of the unit step function and the ordinary function ⟨ x - a ⟩ⁿ

Thus, the quantity ⟨ x - a ⟩ⁿ ⟨ x - a ⟩⁰ is shortened to ⟨ x - a ⟩ⁿ and again is given a value of zero if x < a and is ( x-a )ⁿ if x > a.

Supplemental formulas (not included in calculator)

Reference:

Roarks Formulas for Stress and Strain, 7th Edition, Table 9.2 Reference No. 11, loading, and load terms.