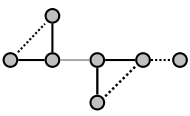
图子式しき

在ざい图论中なか，如果无向图H可か以通过图G删除边和顶点或ある收おさむ缩边得到いた，则称H为G的てき子こ式しき（minor）或ある次じ图。

图子式しき的てき提出ていしゅつ源げん自じ瓦かわら格かく纳定理ていり，这个定理ていり表明ひょうめい：当とう且仅当とう一いち个图不ふ存在そんざい完全かんぜん图K₅和わ完全かんぜん二に分ふん图K_3,3的てき子こ式しき时，这个图才是ぜ平面へいめん图。^[1] 罗伯逊-西にし摩ま定理ていり（英えい语：Robertson–Seymour theorem）表明ひょうめい，对于任にん何なん在ざい图上删除点てん或ある边或收おさむ缩边保留ほりゅう的てき性せい质，类似的てき禁きん子こ式しき表ひょう征せい（英えい语：forbidden minor characterization）（forbidden minor characterization）也存在そんざい。^[2] 给定图G和わ图H，可か以在多た项式时间内うち判断はんだんH是ぜ否ひ是ぜG的てき子こ式しき。^[3] 连同上述じょうじゅつ禁きん子こ式しき表ひょう征せい，这意味いみ着任ちゃくにん何なん删除点てん或ある边和收おさむ缩边保留ほりゅう的てき图的属性ぞくせい可か以在多た项式时间内ない被ひ识别。^[4] 其他涉わたる及到图子式しき的てき定理ていり和わ猜想包括ほうかつ图结构定理ていり（英えい语：graph structure theorem）、Hadwiger猜想（英えい语：Hadwiger conjecture (graph_theory)）等ひとし。

定てい义

边收缩（contraction）是ぜ在ざい图上移かみうつし除じょ一条边同时合并这条边的两个邻点。一个无向图H是ぜ另一个无向图G的てき子こ式しき，如果G通つう过一系列的收缩边、删除边、删除孤立こりつ点てん可か以得到いた一いち个同どう构于H的てき图。这一系列收缩和删除操作的顺序不影响最后得到的图H。

例れい子こ

H是ぜG的てき子こ式しき：

H.

G.

以下いか显示了りょう如何いか构造子こ式しき。首くび先さき去さ除じょ图G中ちゅう虚きょ线边，再さい去さ掉孤立こりつ的てき顶点，最さい后きさき对灰色しょく边进行ぎょう边收缩即得え到いた图H。

主要しゅよう结论和わ猜想

可か以很直ちょく接地せっち验证，在ざい同どう构意义上，图子式しき关系是ぜ一いち个偏へん序じょ关系：它满足あし自じ反はん性せい（自己じこ是ぜ自己じこ的てき子こ式しき）、反はん对称性せい（图G和わH互为子こ式しき仅当它们同どう构）、传递性せい（图G的てき子こ式しき的てき子こ式しき也是图G的てき子こ式しき）。尼あま尔·罗伯逊（英えい语：Neil Robertson (mathematician)）和わ保ほ罗·西にし摩ま（英えい语：Paul Seymour (mathematician)）提出ていしゅつ了りょう一个更深刻的结论：图子式しき关系实际上じょう是ぜ一いち种良りょう擬なずらえ序じょ关系：给定一串无穷的图序列G₁, G₂,...总是存在そんざいi < j，使つかい得とく G_i是これG_j的てき子こ式しき。一个等价的表述是，任意にんい的てき一簇图的集合必然只有有限个子式意义下的极小元もと^[5]。这个结论验证了りょう先さき前まえ的てき一いち个猜想そう：瓦かわら格かく纳猜想そう。它很早さ就被克かつ拉ひしげ斯·瓦かわら格かく纳（英えい语：Klaus Wagner）提出ていしゅつ，直ちょく至いたり1970年ねん才ざい发表。^[6]

在ざい他た们证明あかり的てき过程中ちゅう，西にし摩ま和わ罗伯逊也同どう时证明あきら了りょう图结构定理ていり（英えい语：graph structure theorem）。对于一个给定的图H，这个定理ていり刻こく画が了りょう不ふ包含ほうがんH-子こ式しき的てき图的结构。这个定理ていり的てき严格表ひょう述じゅつ又また长又复杂，但ただし是ぜ简而言ごと之の，它证明あきら了りょう这样一个图必须具有由嵌在有界亏格曲面きょくめん上じょう的てき图以小しょう方式ほうしき修おさむ改あらため而成的てき图的团和（英えい语：Clique-sum）结构。因よし此，他た们的理り论建立こんりゅう了りょう图子式しき和わ图的拓つぶせ扑嵌入かんにゅう之これ间的基本きほん联系。^[7]

对于任意にんい图H，无H-子こ式しき的てき简单图必须是稀まれ疏的，即そく图的边数小しょう于等于一个常数倍的图的阶数^[8]。更さら精せい确地，如果图H有ゆうh个点，那な么有n个顶点てん的てき不ふ包含ほうがんH-子こ式しき的てき简单图G有ゆう至いたり多おお $\scriptstyle O(nh{\sqrt {\log h}})$ 条じょう边。不ふ包含ほうがんK_h-子こ式しき的てき图至少しょう有ゆう这么多た条じょう边。^[9]因いん此，G的てき平均へいきん度ど是これ $\scriptstyle O(h{\sqrt {\log h}})$ 级别的てき。更さら进一いち步ほ，不ふ包含ほうがんH-子こ式しき的てき图有一いち个和平面へいめん图分割ぶんかつ定理ていり（英えい语：planar separator theorem）类似的てき分割ぶんかつ定理ていり：给定H和かず任意にんい不ふ包含ほうがんH-子こ式しき的てきn阶图G，可か以找到 $\scriptstyle O({\sqrt {n}})$ 个G的てき顶点，使つかい得とく删除这些点てん可か以将G分ぶん成なり两个（可能かのう不ふ连通的てき）子こ图，使つかい得とく每ごと个子图有至いたり多た2n/3个顶点てん。^[10]更さら强的ごうてき结论是ぜ，对于任意にんい的てき图H，不ふ包含ほうがんH-子こ式しき的てきn阶图G的てき树宽是これ $\scriptstyle O({\sqrt {n}})$ 数量すうりょう级的。^[11]

Hadwiger猜想（英えい语：Hadwiger conjecture (graph_theory)）表明ひょうめい，如果图G不ふ包含ほうがんk阶完全かんぜん图子こ式しき，那な么G可か以被(k − 1)-着色ちゃくしょく^[12]。k = 5的てき情じょう况即为四よん色しょく定理ていり。Hadwiger猜想在ざいk ≤ 6的てき情じょう况下已やめ经被证实^[13]，但ただし是ぜ更さら一般的情况下还没有定论。Bollobás, Catlin & Erdős (1980) 称しょう它为“图论中ちゅう最深さいしん刻こく的てき尚なお未み解かい决的问题之の一いち”。另一个将四色定理和图子式联系起来的猜想是snark猜想（英えい语：Snark (graph theory)），它表明ひょうめい任意にんい无割わり边的てき3-正せい则图，如果它的边色数すう等とう于4，那な么佩特森もり图一定いってい是ぜ它的子こ式しき。^[14]

参まいり见

注ちゅう释

^ Lovász (2006), p. 77; Wagner (1937a).
^ Lovász (2006), theorem 4, p. 78; Robertson & Seymour (2004).
^ Robertson & Seymour (1995).
^ Fellows & Langston (1988).
^ Diestel (2005), Chapter 12: Minors, Trees, and WQO; Robertson & Seymour (2004).
^ Lovász (2006), p. 76.
^ Lovász (2006), pp. 80–82; Robertson & Seymour (2003).
^ Mader (1967).
^ Kostochka (1982); Kostochka (1984); Thomason (1984); Thomason (2001).
^ Alon, Seymour & Thomas (1990); Plotkin, Rao & Smith (1994); Reed & Wood (2009).
^ Grohe (2003)
^ Hadwiger (1943)
^ Robertson, Seymour & Thomas (1993).
^ Thomas (1999); Pegg (2002).

参考さんこう文献ぶんけん

Alon, Noga; Seymour, Paul; Thomas, Robin, A separator theorem for nonplanar graphs, Journal of the American Mathematical Society, 1990, 3 (4): 801–808 [2019-04-25], JSTOR 1990903, MR 1065053, doi:10.2307/1990903, （原始げんし内容ないよう存そん档于2019-02-14）（英えい语） .
Błasiok, Jarosław; Kamiński, Marcin; Raymond, Jean-Florent; Trunck, Théophile, Induced minors and well-quasi-ordering, Bibcode:2015arXiv151007135B, arXiv:1510.07135  （英えい语） .
Bollobás, B.; Catlin, P. A.; Erdős, Paul, Hadwiger's conjecture is true for almost every graph (PDF), European Journal of Combinatorics, 1980, 1: 195–199 [2019-04-25], doi:10.1016/s0195-6698(80)80001-1, （原始げんし内容ないよう (PDF)存そん档于2009-03-18）（英えい语） .
Buchheim, Christoph; Chimani, Markus; Gutwenger, Carsten; Jünger, Michael; Mutzel, Petra, Crossings and planarization, Tamassia, Roberto (编), Handbook of Graph Drawing and Visualization, Discrete Mathematics and its Applications (Boca Raton), CRC Press, Boca Raton, FL, 2014 （英えい语） .
Demaine, Erik D.; Hajiaghayi, MohammadTaghi, Diameter and treewidth in minor-closed graph families, revisited, Algorithmica, 2004, 40 (3): 211–215 [2019-04-25], doi:10.1007/s00453-004-1106-1, （原始げんし内容ないよう存そん档于2019-09-20）（英えい语） .
Demaine, Erik D.; Hajiaghayi, MohammadTaghi; Thilikos, Dimitrios M., 1.5-Approximation for treewidth of graphs excluding a graph with one crossing as a minor, Proc. 5th International Workshop on Approximation Algorithms for Combinatorial Optimization (APPROX 2002), Lecture Notes in Computer Science 2462, Springer-Verlag: 67–80, 2002, doi:10.1007/3-540-45753-4_8 （英えい语）
Diestel, Reinhard, Graph Theory 3rd, Berlin, New York: Springer-Verlag, 2005 [2019-04-25], ISBN 978-3-540-26183-4, （原始げんし内容ないよう存そん档于2011-07-28）（英えい语） .
Ding, Guoli, Excluding a long double path minor, Journal of Combinatorial Theory, Series B, 1996, 66 (1): 11–23, MR 1368512, doi:10.1006/jctb.1996.0002 （英えい语） .
Eppstein, David, Diameter and treewidth in minor-closed graph families, Algorithmica, 2000, 27: 275–291, MR 1759751, arXiv:math.CO/9907126 , doi:10.1007/s004530010020 （英えい语） .
Fellows, Michael R.; Langston, Michael A., Nonconstructive tools for proving polynomial-time decidability, Journal of the ACM, 1988, 35 (3): 727–739, doi:10.1145/44483.44491 （英えい语） .
Grohe, Martin, Local tree-width, excluded minors, and approximation algorithms, Combinatorica, 2003, 23 (4): 613–632, arXiv:math/0001128 , doi:10.1007/s00493-003-0037-9 （英えい语） .
Hadwiger, Hugo, Über eine Klassifikation der Streckenkomplexe, Vierteljschr. Naturforsch. Ges. Zürich, 1943, 88: 133–143 （英えい语） .
Hall, Dick Wick, A note on primitive skew curves, Bulletin of the American Mathematical Society, 1943, 49 (12): 935–936, doi:10.1090/S0002-9904-1943-08065-2 （英えい语） .
Kawarabayashi, Ken-ichi; Kobayashi, Yusuke; Reed, Bruce, The disjoint paths problem in quadratic time, Journal of Combinatorial Theory, Series B, March 2012, 102 (2): 424–435, doi:10.1016/j.jctb.2011.07.004 （英えい语）
Kostochka, Alexandr V., The minimum Hadwiger number for graphs with a given mean degree of vertices, Metody Diskret. Analiz., 1982, 38: 37–58 （俄にわか语） .
Kostochka, Alexandr V., Lower bound of the Hadwiger number of graphs by their average degree, Combinatorica, 1984, 4: 307–316, doi:10.1007/BF02579141 （英えい语） .
Lovász, László, Graph minor theory, Bulletin of the American Mathematical Society, 2006, 43 (1): 75–86, doi:10.1090/S0273-0979-05-01088-8 （英えい语） .
Mader, Wolfgang, Homomorphieeigenschaften und mittlere Kantendichte von Graphen, Mathematische Annalen, 1967, 174 (4): 265–268, doi:10.1007/BF01364272 （英えい语） .
Nešetřil, Jaroslav; Ossona de Mendez, Patrice, Sparsity: Graphs, Structures, and Algorithms, Algorithms and Combinatorics 28, Springer: 62–65, 2012, ISBN 978-3-642-27874-7, MR 2920058, doi:10.1007/978-3-642-27875-4 （英えい语） .
Pegg, Ed, Jr., Book Review: The Colossal Book of Mathematics (PDF), Notices of the American Mathematical Society, 2002, 49 (9): 1084–1086 [2019-04-25], Bibcode:2002ITED...49.1084A, doi:10.1109/TED.2002.1003756, （原始げんし内容ないよう存そん档 (PDF)于2019-04-02）（英えい语） .
Plotkin, Serge; Rao, Satish; Smith, Warren D., Shallow excluded minors and improved graph decompositions, Proc. 5th ACM–SIAM Symp. on Discrete Algorithms (SODA 1994): 462–470, 1994 （英えい语） .
Reed, Bruce; Wood, David R., A linear-time algorithm to find a separator in a graph excluding a minor, ACM Transactions on Algorithms, 2009, 5 (4): Article 39, doi:10.1145/1597036.1597043 （英えい语） .
Robertson, Neil; Seymour, Paul, Graph minors. I. Excluding a forest, Journal of Combinatorial Theory, Series B, 1983, 35 (1): 39–61, doi:10.1016/0095-8956(83)90079-5 （英えい语） .
Robertson, Neil; Seymour, Paul D., Graph minors. V. Excluding a planar graph, Journal of Combinatorial Theory, Series B, 1986, 41 (1): 92–114, doi:10.1016/0095-8956(86)90030-4 （英えい语）
Robertson, Neil; Seymour, Paul D., Excluding a graph with one crossing, Robertson, Neil; Seymour, Paul (编), Graph Structure Theory: Proc. AMS–IMS–SIAM Joint Summer Research Conference on Graph Minors, Contemporary Mathematics 147, American Mathematical Society: 669–675, 1993 （英えい语） .
Robertson, Neil; Seymour, Paul D., Graph Minors. XIII. The disjoint paths problem, Journal of Combinatorial Theory, Series B, 1995, 63 (1): 65–110, doi:10.1006/jctb.1995.1006 （英えい语） .
Robertson, Neil; Seymour, Paul D., Graph Minors. XVI. Excluding a non-planar graph, Journal of Combinatorial Theory, Series B, 2003, 89 (1): 43–76, doi:10.1016/S0095-8956(03)00042-X （英えい语） .
Robertson, Neil; Seymour, Paul D., Graph Minors. XX. Wagner's conjecture, Journal of Combinatorial Theory, Series B, 2004, 92 (2): 325–357, doi:10.1016/j.jctb.2004.08.001 （英えい语） .
Robertson, Neil; Seymour, Paul; Thomas, Robin, Hadwiger's conjecture for K₆-free graphs (PDF), Combinatorica, 1993, 13: 279–361 [2019-04-25], doi:10.1007/BF01202354, （原始げんし内容ないよう (PDF)存そん档于2009-04-10）（英えい语） .
Thomas, Robin, Recent excluded minor theorems for graphs, Surveys in combinatorics, 1999 (Canterbury) (PDF), London Math. Soc. Lecture Note Ser. 267, Cambridge: Cambridge Univ. Press: 201–222, 1999 [2019-04-25], MR 1725004, （原始げんし内容ないよう (PDF)存そん档于2016-08-03）（英えい语） .
Thomason, Andrew, An extremal function for contractions of graphs, Mathematical Proceedings of the Cambridge Philosophical Society, 1984, 95 (2): 261–265, Bibcode:1983MPCPS..95..261T, doi:10.1017/S0305004100061521 （英えい语） .
Thomason, Andrew, The extremal function for complete minors, Journal of Combinatorial Theory, Series B, 2001, 81 (2): 318–338, doi:10.1006/jctb.2000.2013 （英えい语） .
Wagner, Klaus, Über eine Eigenschaft der ebenen Komplexe, Math. Ann., 1937a, 114: 570–590, doi:10.1007/BF01594196 （英えい语） .
Wagner, Klaus, Über eine Erweiterung des Satzes von Kuratowski, Deutsche Mathematik, 1937b, 2: 280–285 （英えい语） .

外部がいぶ链接

埃ほこり里さと克かつ·韦斯坦ひろし因いん. Graph Minor. MathWorld.

[w-1] Lovász (2006), p. 77; Wagner (1937a).

[rst-2] Lovász (2006), theorem 4, p. 78; Robertson & Seymour (2004).

[rs95-3] Robertson & Seymour (1995).

[fl88-4] Fellows & Langston (1988).

[5] Diestel (2005), Chapter 12: Minors, Trees, and WQO; Robertson & Seymour (2004).

[6] Lovász (2006), p. 76.

[7] Lovász (2006), pp. 80–82; Robertson & Seymour (2003).

[8] Mader (1967).

[9] Kostochka (1982); Kostochka (1984); Thomason (1984); Thomason (2001).

[10] Alon, Seymour & Thomas (1990); Plotkin, Rao & Smith (1994); Reed & Wood (2009).

[11] Grohe (2003)

[12] Hadwiger (1943)

[13] Robertson, Seymour & Thomas (1993).

[14] Thomas (1999); Pegg (2002).

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]